Minimum steel, love it or hate it, we have minimums for a reason even if it seems like overkill sometimes given that we may have very low demands.
This post will demonstrate why (I hope) we are required to comply with these arbitrary limits. Usually I’m going into these posts with only the theoretical background of why something exists, but hopefully once we get into it this theory can actually be demonstrated.
Minimum reinforcement is usually based on a formula that contains a concrete strength amoung other properties being used. The concrete strength is usually specified in terms of the lower 5% characteristic 28 day strength. This is a minimum value used for design purposes, intended to give a minimum dependable strength.
In New Zealand these minimum reinforcement provisions are the maximum of:-
![]() or
or ![]()
In the real world however, concrete gains strength over time. Reaching upwards of 1.5 to 2.0 times the minimum design value under longer term conditions.
Now one thing to note is that the concrete suppliers already aim for a higher strength in their mix design than the specified 28 day compressive strength. This is so in the worst cases they still meet the specified minimum strength for the supplied concrete. So, you’ve almost always got 5-10MPa additional concrete strength right from the get-go.
This means the true strength of an uncracked concrete section is enhanced from the design values due to this moderate to reasonably considerable increase in concrete strength over time.
There’s also a small increase in the reinforced moment capacity due to this increase, but for the most part the reinforced section capacity at minimum levels of reinforcement is relatively insensitive to the concrete strength used.
A minimum percentage of reinforcement is intended to ensure that should a section crack, then the reinforced capacity should be higher than the cracking moment capacity of a section.
This provision is intended to ensure a degree of ductility is possible. Driving further distributed cracking as the concrete section capacity is in fact weaker than the reinforced section capacity. Essentially forcing the yielding of the reinforcing to occur over a longer length of bar. This equates to more opportunity for energy dissipation, leading to a more dependable ductile response.
If you don’t have this hierarchy, you have the potential to get a single crack, this is bad. The reason being because the reinforced moment capacity is weaker than the cracking moment, the reinforcement crossing the single crack sees all of the strain hardening that is occurring. Strain it far enough (particularly under cyclic seismic loading), and you’ll fracture the bars due to low cycle fatigue. Fracturing bars during an earthquake is bad like I mentioned earlier for obvious reasons!
This very effect was observed in a number of structures during the 2011 earthquakes here in New Zealand. Particularly in walls with close to minimum reinforcement, singularly or doubly reinforced. Single cracks formed, and the vertical reinforcing in the wall ends progressively fractured during successive cycles of shaking. In walls part of the issue is that the axial load affects the cracking moment capacity of the section, and the exact level of axial load is really unknown in practice.
For walls in New Zealand at least, this issue was addressed by a subsequent code revision by doubling the minimum reinforcement content in the end regions of walls!
That’s how you deal with a perceived code issue. Double the requirement, no ones really going to argue with you that way, job done. No changes occurred for any other provisions for beams or columns though, there was some talk of it being changed at one point. But nothing emerged in the draft or final version of amendment #3 to NZS3101.
So we’ll look at this scenario in particular. Seeing if the provisions in the New Zealand concrete standard adequately protect against this phenomenon and achieve the hierarchy required, especially in consideration of the real world strength gains going on with the concrete.
So we’ll undertake the following:-
- Determine the minimum reinforcement for an arbitrary rectangular beam section based on the design concrete strength in accordance with NZS3101:2006. Assuming
 .
. - Determine the concrete cracking moment based on a range of long term strength gains, say 1.0 to 2.0 times the design concrete strength.
- Lastly compare the member capacity achieved based on minimum reinforcement being provided in that section and the concrete cracking moment capacity. See if we have a hierarchy in so far as the reinforced capacity is higher than the concrete cracking capacity.
Won’t bother you with the details of the calculations as it’s not exactly rocket science. Results below :-
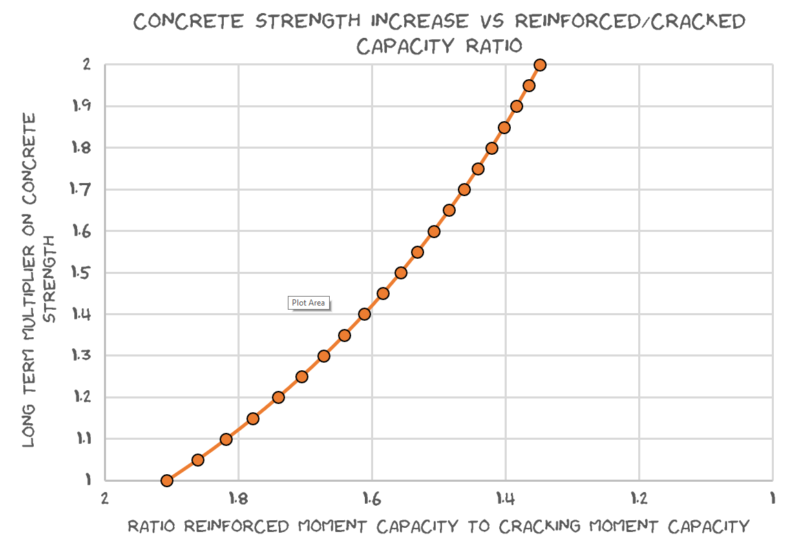
So explaining the graph…. What we are seeing is if the concrete is right on the money at the minimum 28 day specified strength, then the minimum reinforcement based reinforced section capacity is about 1.9 times the cracking moment.
As the real concrete strength increases over and above the minimum 28 day specified strength, then we start to see this ratio of strength over and above the cracking moment capacity being eroded. If we were to get to 2 times the minimum 28 day strength, then our 1.9 ratio drops to about 1.35.
Conclusion
So basically, the code seems to achieve the required hierarchy which is expected (always good when these things work out). Keep in mind this was for a rectangular member. This result would hopefully be similar for other shapes (circular/tee sections for example), I’ll leave that to you to evaluate.
So hopefully if you’re going to take away one thing from all this carry on, you’ll take away the reason for the minimum reinforcement provision above anything else. So no more sneaking designs out under the limits like the sheep out there!
Thanks for another interesting post! I like the way you use calculations in your explanations.